Riemann sums are used to estimate the area under a curve by adding small, rectangular slices. These sums serve as the foundation for integrals. Understanding how the limit of a Riemann sum relates to a definite integral is a key concept in AP® Calculus AB-BC.

Therefore, this post explains how to set up a Riemann sum and then see why it converges to a definite integral. This knowledge is essential for anyone wanting to move from summation notation to definite integral notation.

What Is a Riemann Sum?

Defining Subintervals and Partitions

To begin, consider an interval [a, b] on the x-axis. Divide this interval into n smaller sections called subintervals. Each subinterval has a width labeled \Delta x.

Therefore, if the interval [a, b] has length b - a, then:

\Delta x = \frac{b - a}{n}

Next, write all subintervals as:

[a, a + \Delta x], [a + \Delta x, a + 2\Delta x], \dots, [a + (n-1)\Delta x, b]

Evaluating the Function on Each Subinterval

Within each subinterval, choose a sample point x_i. This point can be at the left end, right end, or somewhere in the middle of the subinterval. Then, calculate the function value f(x_i).

Next, multiply f(x_i) by the width \Delta x. This product represents the area of one rectangle whose height is f(x_i) and base is \Delta x.

Summation

After finding f(x_i) \Delta x for each subinterval, sum all those products. The Riemann sum is:

\sum_{i=1}^{n} f(x_i) \Delta x

As n gets larger, \Delta x gets smaller. Therefore, the total sum becomes more accurate.

Understanding the Limit of a Riemann Sum

Convergence to the Definite Integral

When the number of subintervals increases toward infinity, \Delta x \rightarrow 0. In that limit, the Riemann sum provides the exact area under f(x) from x=a to x=b. This idea is crucial for recognizing that:

\lim_{n \to \infty} \sum_{i=1}^n f(x_i^*) \Delta x = \text{Exact Area}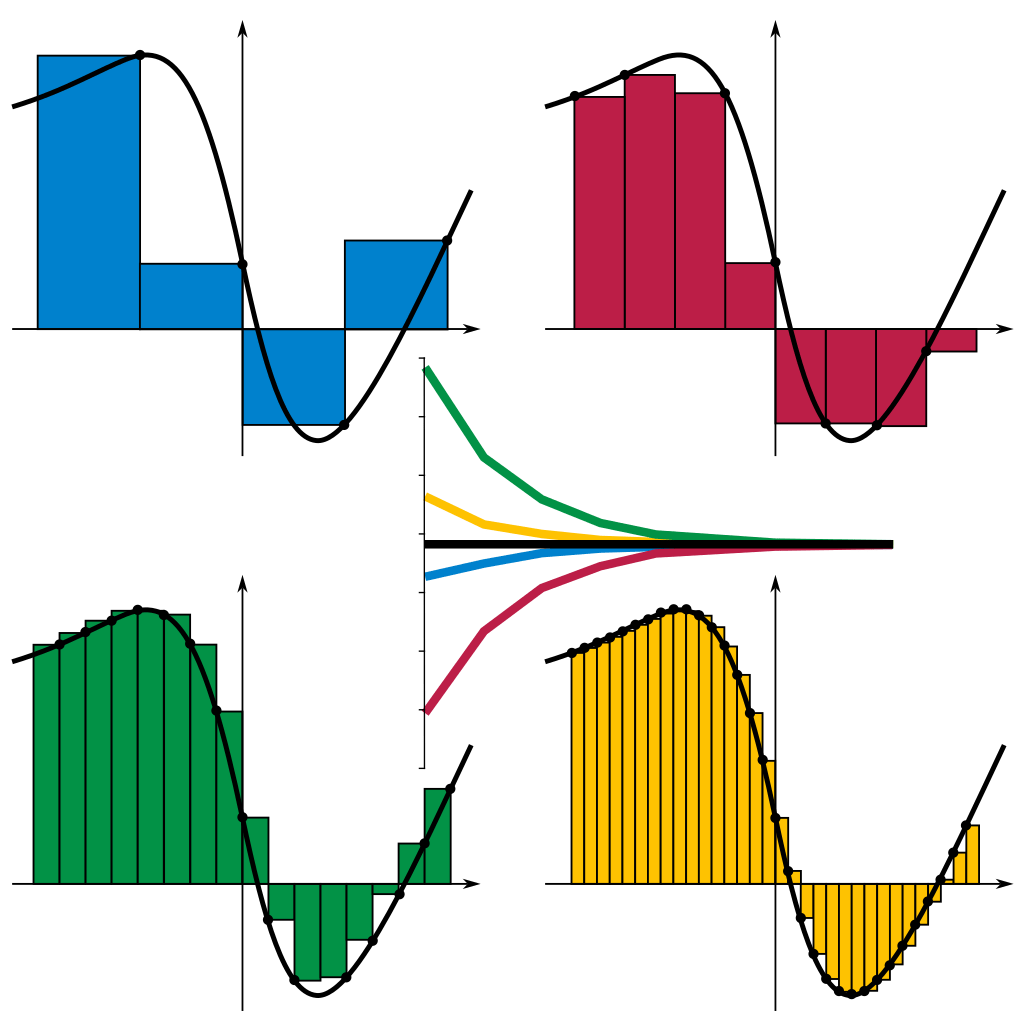

Definite Integral Notation

The definite integral notation connects directly to this limit. It is written as:

\int_{a}^{b} f(x) dx

Hence, the integral symbol \int can be viewed as a “fancy S,” which stands for summation. The expression \int_{a}^{b} f(x) , dx exactly equals the limit of the Riemann sum.

Converting a Riemann Sum to a Definite Integral

Converting a Riemann sum to a definite integral involves replacing \sum_{i=1}^{n} f(x_i^*) \Delta x with \int_{a}^{b} f(x) , dx in the limit as n \rightarrow \infty.

Therefore, if a problem begins in summation notation, apply the limit process. Then rewrite with the integral sign and the correct bounds. This step is central in AP® Calculus when moving from a Riemann sum to integral notation.

Example: Riemann Sum to Definite Integral

Write a definite integral equivalent to the following limit.

\lim\limits_{n \to \infty} \sum_{i=1}^n \left( \cos\left( \dfrac{2i}{n} \right) \cdot \dfrac{3}{n} \right)

We are given the limit:

\lim\limits_{n \to \infty} \sum_{i=1}^n \left( \cos\left( \dfrac{2i}{n} \right) \cdot \dfrac{3}{n} \right)

This is a Riemann sum of the form:

\lim\limits_{n \to \infty} \sum_{i=1}^n f(x_i^*) \cdot \Delta x

Identify \Delta x

The \Delta x term is the constant multiplier outside the function, which in this case is:

\Delta x = \dfrac{3}{n}

Determine the interval

Since \Delta x = \dfrac{b - a}{n}, and \Delta x = \dfrac{3}{n}, it must be that:

b - a = 3

This means the interval has length 3. If we assume the first subinterval starts at x = 0, then:

a = 0,\quad b = 3

Identify x_i^*

In the sum, we see:

\cos\left( \dfrac{2i}{n} \right)

We want to express this in terms of x_i^* = a + i \cdot \Delta x. From Step 2, we know:

x_i^* = 0 + i \cdot \dfrac{3}{n} = \dfrac{3i}{n}

So we want:

\cos\left( \dfrac{2i}{n} \right) = f(x_i^*) = f\left( \dfrac{3i}{n} \right)

This means the function is:

f(x) = \cos\left( \dfrac{2}{3}x \right)

Write the equivalent definite integral

Now we can rewrite the Riemann sum as a definite integral:

\int_0^3 \cos\left( \dfrac{2}{3}x \right) \, dx

So the final answer is:

\int_0^3 \cos\left( \dfrac{2}{3}x \right) \, dx

Quick Reference Chart (Vocabulary and Definitions)

Term	Definition/Explanation
Riemann Sum	A sum of function values times subinterval widths that approximates the area under a curve.
Subinterval	A smaller division of the interval [a, b].
\Delta x	The width of each subinterval.
Sample Point (x_i^*)	A point in each subinterval at which the function value is found.
Definite Integral	The limit of Riemann sums, representing the exact area under f(x) between x = a and x = b.

Conclusion

In summary, the limit of a Riemann sum reveals the precise area under f(x). This is exactly what the definite integral represents. Consequently, definite integral notation is a compact way to show the result of adding infinitely many small pieces under a curve.

Finally, converting a Riemann sum to a definite integral is an essential skill for advanced calculus topics. Mastery of this connection between a finite sum and its limit as n \to \infty will pave the way for applying the Fundamental Theorem of Calculus and beyond.

Sharpen Your Skills for AP® Calculus AB-BC

Are you preparing for the AP® Calculus exam? We’ve got you covered! Try our review articles designed to help you confidently tackle real-world math problems. You’ll find everything you need to succeed, from quick tips to detailed strategies. Start exploring now!

Need help preparing for your AP® Calculus AB-BC exam?

Albert has hundreds of AP® Calculus AB-BC practice questions, free responses, and an AP® Calculus AB-BC practice test to try out.