Understanding whether a series converges is crucial in calculus and other fields. Convergence means that adding infinitely many terms leads to a finite result, which can be vital in scientific research, engineering, and advanced math. Therefore, learning the integral test for convergence and knowing when to apply it is an essential skill.
What We Review
What Is the Integral Test for Convergence?
The integral test for convergence states that if a function f(x) satisfies certain conditions, then the convergence of the series \sum_{n=1}^{\infty} f(n) can be determined by the convergence of the improper integral \int_{1}^{\infty} f(x) dx.
In simpler terms, if the area under the curve of f(x) converges when integrated from 1 to infinity, then the sum of the series \sum_{n=1}^{\infty} f(n) also converges. However, if that improper integral diverges (becomes infinitely large, for example), the series diverges. This approach neatly connects infinite sums to continuous calculus techniques.
Conditions for Validity
To use the integral test for convergence, the function f(x) must meet these criteria on the interval [1, ∞]:
- Positive: f(x) > 0
- Continuous: no gaps or breaks in f(x)
- Monotonically Decreasing: f'(x) \le 0 for x \ge 1
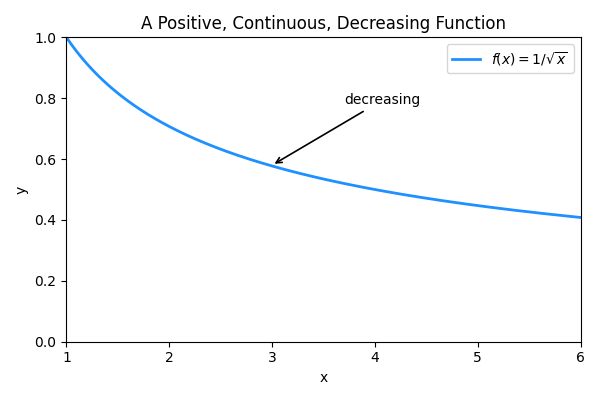
These conditions ensure the comparison between the infinite sum and the improper integral is consistent. When functions violate any of these rules, the integral test might not give accurate results, and a different test could be more suitable.
Conditions for Using the Integral Test
A function must check the following properties before applying the integral test:
- Positivity
- The function’s values must stay above zero for all x \ge 1. If values dip below zero, the integral test can become unreliable, and another method may be necessary.
- Continuity
- Because the integral test relies on integrating the function, the function must not have gaps or discontinuities. Continuous functions let us set up the improper integral without complications.
- Monotonically Decreasing
- The function should never increase on the interval of interest. For x \ge 1, the derivative f'(x) should be less than or equal to zero. This decreasing nature ensures the integral and the discrete sum mirror each other in behavior.
These conditions matter because they uphold the logic behind measuring the area under a curve as a stand-in for an infinite sum. When functions remain positive, continuous, and decreasing, the total area measured by the integral lines up closely with the sum of the series terms.
Step-by-Step Example 1
Consider the series \sum_{n=1}^{\infty} \frac{1}{n^2}. Follow these steps to see why the integral test for convergence concludes that this series converges.
- Identify the corresponding function.
- Let f(x) = \frac{1}{x^2}.
- Verify conditions.
- f(x) is positive for x \ge 1.
- f(x) is continuous for x \ge 1.
- f(x) is monotonically decreasing because its derivative \frac{d}{dx}\left(\frac{1}{x^2}\right) = -\frac{2}{x^3} is less than 0 for x \ge 1.
- Set up the improper integral.
- \int_{1}^{\infty} \frac{1}{x^2} dx
- Evaluate the integral.
- Find the antiderivative: \int \frac{1}{x^2} dx = \int x^{-2} dx = -\frac{1}{x} + C
- Take the limit as the upper bound goes to infinity: \lim_{b \to \infty} \left[ -\frac{1}{x} \right]_{1}^{b} = \lim_{b \to \infty} \left(-\frac{1}{b} + \frac{1}{1}\right) = 0 + 1 = 1
- Conclude convergence.
- Because the improper integral converges to 1, the integral test for convergence states that \sum_{n=1}^{\infty} \frac{1}{n^2} converges.
Step-by-Step Example 2
Next, examine the series \sum_{n=1}^{\infty} \frac{1}{n} (the harmonic series). The integral test for convergence will show a different result.
- Identify the corresponding function.
- Let f(x) = \frac{1}{x}.
- Verify conditions.
- f(x) is positive for x \ge 1.
- f(x) is continuous for x \ge 1.
- f(x) is monotonically decreasing because its derivative \frac{d}{dx}\left(\frac{1}{x}\right) = -\frac{1}{x^2} is less than 0 for x \ge 1.
- Set up the improper integral.
- \int_{1}^{\infty} \frac{1}{x} dx
- Evaluate the integral.
- Find the antiderivative:\int \frac{1}{x} dx = \ln |x| + C
- Take the limit as the upper bound goes to infinity: \lim_{b \to \infty} \left[ \ln|x| \right]_{1}^{b} = \lim_{b \to \infty} (\ln b - \ln 1) = \lim_{b \to \infty} \ln b = \infty
- Conclude divergence.
- Because this improper integral diverges to infinity, the integral test for convergence implies that \sum_{n=1}^{\infty} \frac{1}{n} also diverges.
Common Pitfalls and Misconceptions
- Forgetting to check if the function is decreasing: Always verify that f'(x) \le 0 for x \ge 1. If the function is not decreasing, the conclusions from the integral test for convergence might fail.
- Misinterpreting the integral result: A large integral value does not immediately guarantee divergence. Instead, pay attention to whether it settles to a finite limit or heads to infinity.
- Mixing up tests: At times, a comparison test or ratio test might be easier or more direct. The integral test is often used when it is simpler to integrate the function than to apply other methods.
Quick Reference Chart
| Vocabulary Term | Definition |
| Integral Test for Convergence | A method to determine the convergence or divergence of an infinite series by comparing it to an improper integral. |
| Positive Function | A function f(x) > 0 for x above a certain point. |
| Continuous Function | A function with no breaks or gaps on its domain. |
| Monotonically Decreasing | A function f(x) for which f'(x) \le 0 so it never increases. |
| Convergence | When the sum of the infinite terms of a series settles to a finite number. |
| Divergence | When the sum of the infinite terms of a series grows without bound or fails to reach a finite limit. |
Conclusion
The integral test for convergence links infinite series to improper integrals by checking whether a function is positive, continuous, and monotonically decreasing. If these conditions hold, it is possible to evaluate the associated improper integral to infer convergence or divergence. This test is central in understanding series behavior in AP® Calculus (LIM-7.A.6) and beyond.
Consistent practice with these examples will strengthen problem-solving skills. Whether dealing with classic series, such as \sum \frac{1}{n^p}, or exploring new applications, remember to verify each condition. In many cases, performing the integral test for convergence gives a clear answer to whether a series converges or diverges.
Sharpen Your Skills for AP® Calculus AB-BC
Are you preparing for the AP® Calculus exam? We’ve got you covered! Try our review articles designed to help you confidently tackle real-world math problems. You’ll find everything you need to succeed, from quick tips to detailed strategies. Start exploring now!
Need help preparing for your AP® Calculus AB-BC exam?
Albert has hundreds of AP® Calculus AB-BC practice questions, free responses, and an AP® Calculus AB-BC practice test to try out.