Continuity is a key concept in AP® Calculus AB-BC. It helps in understanding how functions behave without breaks or sudden jumps. Specifically, knowing how to tell if a function is continuous is essential for success on exams and for deeper topics like limits, derivatives, and integrals.
Continuity plays an important role in many problem-solving steps. Therefore, 1.12 confirming continuity over an interval often appears in practice exercises and assessments. The discussion here aligns with LIM-2.B.1 and LIM-2.B.2 requirements. This article outlines everything high school students need to identify continuity at a point and over an interval. It also explores how continuity applies to well-known families of functions and why it matters for real-world math applications.
What We Review
Definition of Continuity at a Point
Understanding the Formal Definition
A function f(x) is continuous at x = c if it meets three conditions:
- f(c) must be defined.
- The limit of f(x) as x approaches c must exist.
- That limit must be equal to f(c).
Thus, continuity at a point means f(x) has no gaps or jumps at that x-value.
Example and Step-by-Step Solution
Consider the function f(x) = x^2 - 4, and check continuity at x = 2.
- Check if f(2) is defined: Substitute x = 2 into f(x). f(2) = 2^2 - 4 = 4 - 4 = 0. Hence, f(2) is defined and equals 0.
- Determine \lim_{x \to 2} f(x): For x^2 - 4, rewrite or directly substitute because it is a polynomial. \lim_{x \to 2} (x^2 - 4) = (2^2 - 4) = 0.
- Compare the limit with f(2): The limit as x approaches 2 is 0, and f(2) is also 0. Therefore, \lim_{x \to 2} f(x) = f(2).
Because all three conditions are satisfied, f(x) is continuous at x = 2.
Confirming Continuity Over an Interval
Continuity on an Interval
A function is continuous on an interval if it remains continuous at each point in that interval. According to LIM-2.B.1, open intervals ((a, b)) consider values strictly between a and b. Closed intervals ([a, b]) also require continuity at the endpoints.
For instance, continuity on [a, b] means the function is continuous for every point between a and b, plus the function must not have a discontinuity at a or b.
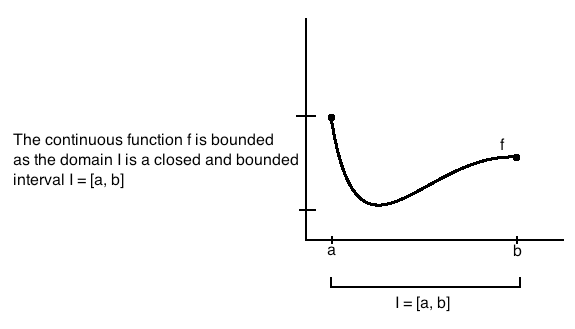
Strategy for Checking Continuity on an Interval
- Identify any points where the function might be undefined.
- Check continuity at those specific points.
- Verify that the rest of the interval has no interruption in function values.
Furthermore, keep an eye out for holes (removable discontinuities), asymptotes, or sudden jumps. These typically occur at points that make a denominator zero or lead to valid limit concerns.
Example and Step-by-Step Solution
Check if the function g(x) = \frac{x + 3}{x - 1} is continuous on the interval (1, \infty).
- Identify points of potential discontinuity: The denominator is zero at x = 1. This suggests a problem at that point.
- Since the interval is (1, \infty), x = 1 is not included. Thus, check continuity for x > 1 only.
- For any x > 1, the denominator (x - 1) is nonzero. Hence g(x) is defined everywhere in that interval.
- Polynomials and rational functions (away from zero denominators) are continuous by their nature. Therefore, g(x) has no gaps for x > 1.
Since there are no points in (1, \infty) that make the denominator zero, g(x) is continuous on (1, \infty).
Families of Continuous Functions
Overview of Common Function Families
There are certain families of functions known for their continuity on their domains. These include:
- Polynomial functions
- Rational functions
- Power functions
- Exponential functions
- Logarithmic functions
- Trigonometric functions
Each family has its own domain rules, yet each is continuous where it is properly defined.
Explanation of Why These Are Continuous on Their Domains
- Polynomials are continuous everywhere because they have no denominators or other complicating factors.
- Rational functions are continuous except where the denominator equals zero.
- Power, exponential, logarithmic, and trigonometric functions each have domains that specify valid x values. Where they are defined, these functions do not have breaks.
Example and Step-by-Step Solution
Consider h(x) = \frac{2x - 4}{x^2 - 1}, which is a rational function.
- Factor the denominator: x^2 - 1 = (x - 1)(x + 1).
- Identify possible discontinuities: When x - 1 = 0 or x + 1 = 0, the function is undefined. That means x = 1 or x = -1.
- Determine the domain: The function is defined for all real x such that x \neq 1 and x \neq -1.
- Conclude continuity on the domain: Because rational functions are continuous on their domain, h(x) is continuous for all x except x = 1 and x = -1.
Hence, outside of those excluded points, h(x) does not experience any jumps or holes. This approach aligns with how to tell if a function is continuous by checking where the function is well-defined.
Why Continuity Matters
Practical Applications
Continuous functions are important in physics, economics, and many other fields. For example, an object’s displacement over time is typically modeled with continuous functions to ensure smooth motion.
Additionally, if a function is not continuous, it might indicate a sudden shift or break in a model. Thus, continuity gives clear insight into predictable and stable behavior.
Connection to Further Topics in Calculus
Continuity also sets the stage for limits and derivatives. The Fundamental Theorem of Calculus (connecting derivatives and integrals) requires functions to be continuous on the relevant intervals. Discontinuities can sometimes be removable, if a single point is the problem, or non-removable, like asymptotes. Recognizing how to pinpoint these issues makes advanced topics easier to master.
Quick Reference Chart of Important Terms and Definitions
| Term | Definition |
| Continuous at a point | A function f is continuous at x = c if f(c) is defined, \lim_{x \to c} f(x) exists, and they are equal. |
| Continuous on an interval | A function is continuous on an interval if it is continuous at every point in that interval. |
| Domain | The set of all possible input values (x-values) for which the function is defined. |
| Polynomial Function | An expression of variables and coefficients using addition, subtraction, multiplication, and non-negative integer exponents. Continuous for all real x. |
| Rational Function | A ratio of two polynomials. Continuous wherever the denominator is not zero. |
| Power Function | A function of the form x^n where n is any real number. Usually continuous if it is defined. |
| Exponential Function | A function of the form a^x with a > 0. Continuous for all real x. |
| Logarithmic Function | The inverse of an exponential function. Continuous for x > 0. |
| Trigonometric Function | Sine, cosine, tangent, etc. Continuous on their domains. |
Conclusion
Continuity ensures a function behaves predictably at every point in its domain. This article showed how to tell if a function is continuous at a single point, as well as 1.12 confirming continuity over an interval. It also covered LIM-2.B.1 and LIM-2.B.2 for AP® Calculus AB-BC, outlining families of common continuous functions and why they matter for advanced topics.
Understanding continuity becomes vital when investigating limits, derivatives, and integrals. Therefore, exploring additional resources and practice problems will strengthen skills involving continuous functions and pave the way for better performance in further calculus studies.
Sharpen Your Skills for AP® Calculus AB-BC
Are you preparing for the AP® Calculus exam? We’ve got you covered! Try our review articles designed to help you confidently tackle real-world math problems. You’ll find everything you need to succeed, from quick tips to detailed strategies. Start exploring now!
Need help preparing for your AP® Calculus AB-BC exam?
Albert has hundreds of AP® Calculus AB-BC practice questions, free responses, and an AP® Calculus AB-BC practice test to try out.








