Integration by parts is a powerful technique that helps in finding antiderivatives of more complex functions. It appears often in AP® Calculus AB-BC and is especially helpful whenever an integrand can be split into two factors. One part should ideally become simpler when differentiated, and the other part should remain manageable when integrated. The method stems from the product rule in differentiation. Throughout this post, you will see several integration by parts examples, including a quick look at tabular integration by parts, and discover when to use integration by parts. Additionally, there will be problems to practice integration by parts, allowing you to build your skills step by step.
What We Review
Understanding the Integration by Parts Formula
Derivation and Intuition
The formula for integration by parts is based on the product rule from differentiation. The product rule states that if f(x) and g(x) are functions of x, then: \frac{d}{dx}\bigl(f(x)g(x)\bigr) = f'(x)g(x) + f(x)g'(x).
By integrating both sides with respect to x, we arrive at: \int f'(x)g(x)dx + \int f(x)g'(x)dx = f(x)g(x) + C.
Rewriting the terms and relabeling f(x) = u and g'(x) = dv, we get the well-known formula: \int udv = uv - \int vdu.
Therefore, this approach is particularly useful when an integrand appears as a product of two simpler parts.
Choosing u and dv
Selecting the right u and dv can make integration easier. One popular guide is “LIATE” (or sometimes “ILATE”), where each letter stands for a function type:
- L: Logarithmic (like \ln(x))
- I: Inverse trigonometric (like \arctan(x))
- A: Algebraic (like x^2)
- T: Trigonometric (like \sin(x), \cos(x))
- E: Exponential (like e^x)
When deciding u, it is often the function that becomes simpler upon differentiation, such as a logarithm or a polynomial. Then, the remaining portion is taken as dv.
Tabular Integration by Parts
Tabular integration by parts is a shortcut for repeated integration by parts. It organizes the steps in a table. On one side, you keep differentiating u until you reach zero (or a simpler expression), and on the other side, you keep integrating dv repeatedly. Then, you alternate signs when summing the products of these terms. This method is particularly effective for integrals involving polynomials multiplied by exponentials or trigonometric functions.
Example: Integrating a Polynomial Times an Exponential Function
Consider the integral:\int x^2 e^x dx.
Step-by-step solution:
- Choose u = x^2. This becomes simpler when differentiated. Therefore, dv = e^x dx.
- Differentiate u: du = 2xdx.
- Integrate dv: v = e^x.
- Apply the integration by parts formula: \int x^2 e^x dx = x^2 e^x - \int e^x \cdot 2x dx.
- Simplify and notice a new integral appears: \int 2x e^x dx. We apply integration by parts again.
- Let u = 2x, so du = 2dx.
- Let dv = e^x dx, so v = e^x.
- Thus, \int 2x e^x dx = 2x e^x - \int 2 e^x dx.
- Finish the final integral: \int 2 e^x dx = 2 e^x.
- Combine all pieces: \int x^2 e^x dx = x^2 e^x - \bigl(2x e^x - 2 e^x\bigr) + C.
- Distribute the negative sign: \int x^2 e^x dx = x^2 e^x - 2x e^x + 2 e^x + C.
- Factor out e^x: \int x^2 e^x dx = e^x(x^2 - 2x + 2) + C.
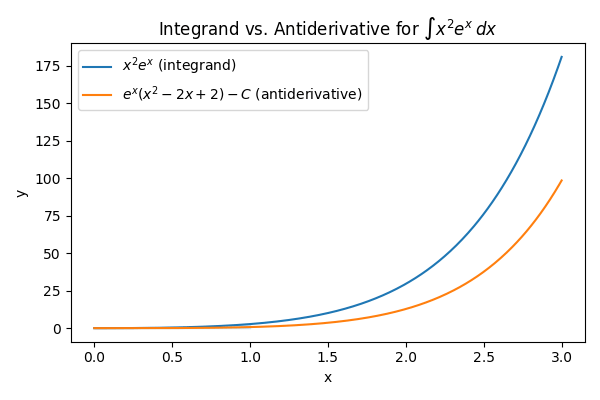
This result shows how integration by parts simplifies what might otherwise be a challenging problem.
Practice Problem: Polynomial and Trigonometric Integration
Try this integral on your own: \int x^2 \cos(x) dx.
Step-by-step solution:
- Choose u = x^2, hence du = 2x dx.
- Let dv = \cos(x)dx. Then v = \sin(x).
- Use the formula: \int x^2 \cos(x) dx = x^2 \sin(x) - \int \sin(x) \cdot 2x dx.
- For \int 2x \sin(x) dx, apply integration by parts again.
- Let u = 2x. Then du = 2 dx.
- Let dv = \sin(x)dx. Then v = -\cos(x).
- So, \int 2x \sin(x) dx = 2x \bigl(-\cos(x)\bigr) - \int -\cos(x) \cdot 2 dx.
- This becomes -2x\cos(x) + 2 \int \cos(x) dx.
- Finish it: = -2x\cos(x) + 2\sin(x).
- Combine it all: \int x^2 \cos(x) dx = x^2 \sin(x) - \Bigl(-2x\cos(x) + 2\sin(x)\Bigr) + C.
- Distribute the negative: = x^2 \sin(x) + 2x\cos(x) - 2\sin(x) + C.
This is the final result.
Common Mistakes and Tips
- Watch out for missing negative signs during the process. However, double-checking each step can prevent such errors.
- Ensure the correct derivative of \ln(x) is used. Therefore, always recall it is \frac{1}{x}.
- Avoid picking a u that gets more complicated upon differentiation. Instead, choose something that simplifies.
- Work on a variety of problems to practice integration by parts, including polynomials with exponentials, trigonometric functions, and logarithmic functions.
Quick Reference Chart
Below is a quick guide for essential terms and steps:
| Term | Definition |
| u | The function chosen for differentiation (often logs, inverse trig, or polynomials). |
| dv | The part of the integrand set aside for integration (often exponentials, trigonometric functions, or the remaining expression). |
| \int u dv = uv - \int v du | The core integration by parts formula. |
| Tabular Integration by Parts | List derivatives of u in one column (until zero or a pattern emerges). List integrals of dv in the next column. Alternate signs (+/–) when summing products diagonally. |
Conclusion
Integration by parts is a cornerstone of calculus methods, especially in AP® Calculus AB-BC. It provides a tool to handle tough integrals that involve products of functions. By choosing u and dv carefully and understanding tabular integration by parts, solving repeated products becomes more systematic. Keep practicing these approaches with problems to practice integration by parts. In time, recognizing when to use integration by parts will become second nature, making more advanced calculus topics much easier to tackle.
Sharpen Your Skills for AP® Calculus AB-BC
Are you preparing for the AP® Calculus exam? We’ve got you covered! Try our review articles designed to help you confidently tackle real-world math problems. You’ll find everything you need to succeed, from quick tips to detailed strategies. Start exploring now!
Need help preparing for your AP® Calculus AB-BC exam?
Albert has hundreds of AP® Calculus AB-BC practice questions, free responses, and an AP® Calculus AB-BC practice test to try out.