Parametric equations are a powerful way to describe curves in the plane. Instead of writing y = f(x), parametric equations define two functions, x(t) and y(t), both in terms of a third variable, often labeled t. Therefore, each value of t provides coordinates (x(t), y(t)) on the curve, which also allows for calculating the second derivative of parametric equations when analyzing curvature and acceleration.
The second derivative of parametric equations is especially important in AP® Calculus AB-BC because it helps reveal information about concavity and inflection points. Hence, finding \frac{d^2y}{dx^2} (the second derivative with respect to x) is a crucial step when analyzing parametric curves. This post explores how to compute the second derivative of a parametric function, explains the formula, and provides practical examples.
What We Review
Parametric Equations Refresher
Defining Parametric Functions
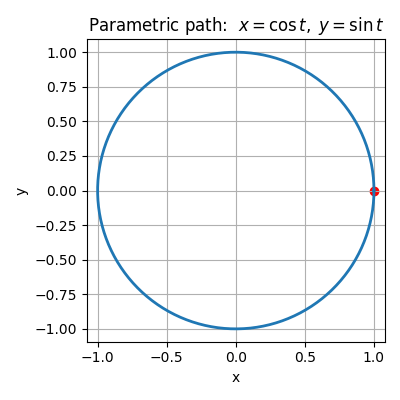
Parametric functions describe x and y using a common parameter t. For instance, x(t) = \cos(t) and y(t) = \sin(t) create a circle. However, unlike simple y=f(x) forms, parametric equations can trace out curves that are not single-valued functions of x. This flexibility allows more complex paths.
Derivative Basics in Parametric Form
The derivatives \frac{dx}{dt} and \frac{dy}{dt} measure how x and y change with respect to t. However, when investigating slope with respect to x, it becomes necessary to use these derivatives to form \frac{dy}{dx}. This concept connects parametric equations to the more familiar idea of a slope from standard calculus courses.
First Derivative dy/dx in Parametric Form
Understanding dy/dx for Parametric Curves
For parametric curves, the slope is found by taking \frac{dy}{dt} and dividing by \frac{dx}{dt}. Thus, the first derivative with respect to x is: \frac{dy}{dx} = \frac{\frac{dy}{dt}}{\frac{dx}{dt}}.It is essential to check that \frac{dx}{dt} \neq 0, otherwise division is not valid.
Example 1: Finding the First Derivative
Consider the parametric equations: x(t) = t^2 and y(t) = t^3 + 1.
Step-by-Step Solution
- Compute \frac{dx}{dt} and \frac{dy}{dt}.
- \frac{dx}{dt} = 2t
- \frac{dy}{dt} = 3t^2
- Form the ratio \frac{dy}{dx} = \frac{3t^2}{2t}.
- Simplify this to \frac{3t^2}{2t} = \frac{3}{2} t, for t \neq 0.
- The result shows that the slope of the tangent line depends on t. Therefore, \frac{3}{2} t is the instantaneous rate of change of y with respect to x at time t.
Introducing the Second Derivative (d²y/dx²)
Why the Second Derivative is Useful
The second derivative of parametric equations helps in determining concavity. When \frac{d^2y}{dx^2} is positive, the curve is concave up. When it is negative, the curve is concave down. Consequently, changes in the sign of the second derivative can show where the curve has inflection points.
Formula for the Second Derivative of Parametric Equations
The standard formula for the second derivative of a parametric function is:
\frac{d^2y}{dx^2} = \frac{\frac{d}{dt}\left(\frac{dy}{dx}\right)}{\frac{dx}{dt}}.Here, \frac{d}{dt}\left(\frac{dy}{dx}\right) denotes the time derivative of the first derivative. Therefore, to find the second derivative of parametric equations, first compute \frac{dy}{dx}, then differentiate that result with respect to t, and finally divide by \frac{dx}{dt}.
Example 2: Finding the Second Derivative
Consider another parametric system:
x(t) = 3t + 1 and y(t) = t^2 - t.Step-by-Step Solution
- Find \frac{dx}{dt} and \frac{dy}{dt}.
- \frac{dx}{dt} = 3
- \frac{dy}{dt} = 2t - 1
- Compute \frac{dy}{dx} = \frac{2t - 1}{3}.
- Each slope value depends on t.
- Differentiate \frac{dy}{dx} with respect to t.
- \frac{d}{dt}\left(\frac{2t - 1}{3}\right) = \frac{2}{3}
- Divide this result by \frac{dx}{dt}.
- Therefore, \frac{d^2y}{dx^2} = \frac{\frac{2}{3}}{3} = \frac{2}{9}.
- The constant \frac{2}{9} implies the curve’s concavity is the same for all t values, since it never changes sign.
Common Pitfalls and Helpful Tips
- Always confirm \frac{dx}{dt} remains nonzero before dividing. Otherwise, the expression for \frac{dy}{dx} becomes undefined.
- Keep track of the sign of the second derivative to discover whether the curve is concave up or concave down.
- Pause to examine critical points where \frac{dx}{dt} = 0, because those points may need a separate investigation.
Quick Reference Chart
| Term | Definition/Explanation |
| Parametric Equation | Functions x(t), y(t) defining a curve |
| \frac{dx}{dt}, \frac{dy}{dt} | Derivatives of x and y with respect to t |
| First Derivative (\frac{dy}{dx}) | \frac{\frac{dy}{dt}}{\frac{dx}{dt}} |
| Second Derivative (\frac{d^2y}{dx^2}) | \frac{\frac{d}{dt}\left(\frac{dy}{dx}\right)}{\frac{dx}{dt}} |
| Concavity | Determined by the sign of the second derivative |
Conclusion
The second derivative of parametric equations offers critical insight into the behavior of a curve. Therefore, calculations of \frac{d^2y}{dx^2} reveal where a curve is bending upward or downward and where an inflection might occur. In AP® Calculus AB-BC, recognizing that the second derivative of a parametric function requires computing \frac{dy}{dx} first is vital. Carefully following the outlined steps makes it easier to avoid errors and effectively analyze any parametric curve.
Sharpen Your Skills for AP® Calculus AB-BC
Are you preparing for the AP® Calculus exam? We’ve got you covered! Try our review articles designed to help you confidently tackle real-world math problems. You’ll find everything you need to succeed, from quick tips to detailed strategies. Start exploring now!
Need help preparing for your AP® Calculus AB-BC exam?
Albert has hundreds of AP® Calculus AB-BC practice questions, free responses, and an AP® Calculus AB-BC practice test to try out.
